-
-
January 25, 2023 at 7:34 am
 FAQParticipant
FAQParticipantWhat is Rigid response ? Details are in the Theory Reference, but the simple explanation is that we try to get a ‘max’ or ‘final’ response in Response Spectrum analyses through mode-combination methods. The mode-combination methods try to account for modes acting together at once. When we have a ‘rigid’ response (high frequencies), the modes are thought to act together (in-phase), so they may be directly added (algebraic sum). On the other hand, if modes may be out-of-phase with each other, we use other approaches (SRSS is the least conservative of these approaches). The Rigid response tries to separate ‘rigid’ and non-rigid (‘periodic’) responses separately. To do this, we have two methods – Gupta and Lindley-Yow. Gupta uses two frequencies – below f1, we don’t algebraically sum; above f2, we algebraically sum, and we vary in-between when f1 < f < f2. Lindley-Yow uses a ratio instead. Once the 'rigid' response is calculated, it is then combined with the 'periodic' response via SRSS. If one wishes to include Rigid Response effect, this does change the mode combination completely (see Equations in Theory Reference cited above) by separating modes into 'rigid' and 'periodic' modes and combining them differently. When to use Rigid response ? To address whether rigid response is needed, one needs to answer this question: Does one want to separate 'periodic' and 'rigid' response for the dynamic behavior of the system? One needs to study the dynamic behavior of the system and understand when the modes are in phase and when do they transition to being out of phase. ANSYS Mechanical offer users multiple mode combination methods to determine the 'peak' response of the system (SRSS, CQC, ROSE, etc.). Are these mode combination methods sufficient for the analysis being performed? If userse understand their system behavior and they understand that, at certain frequencies, the modes should behave in-phase - SRSS or other mode combination methods would not account for this. Therefore, one wants a way to manually specify frequencies at which the modes are summed algebraically, and the Rigid Response method offers such an alternative to standard mode-combination methods. If one doesn't know at which frequencies his/her modes act in-phase, it's probably better not to use rigid response method. If an analyst knows the cut-off at which frequencies their modes act in-phase, that is the frequency they input for Gupta or Lindley-Yow methods.
-


Introducing Ansys Electronics Desktop on Ansys Cloud
The Watch & Learn video article provides an overview of cloud computing from Electronics Desktop and details the product licenses and subscriptions to ANSYS Cloud Service that are...

How to Create a Reflector for a Center High-Mounted Stop Lamp (CHMSL)
This video article demonstrates how to create a reflector for a center high-mounted stop lamp. Optical Part design in Ansys SPEOS enables the design and validation of multiple...
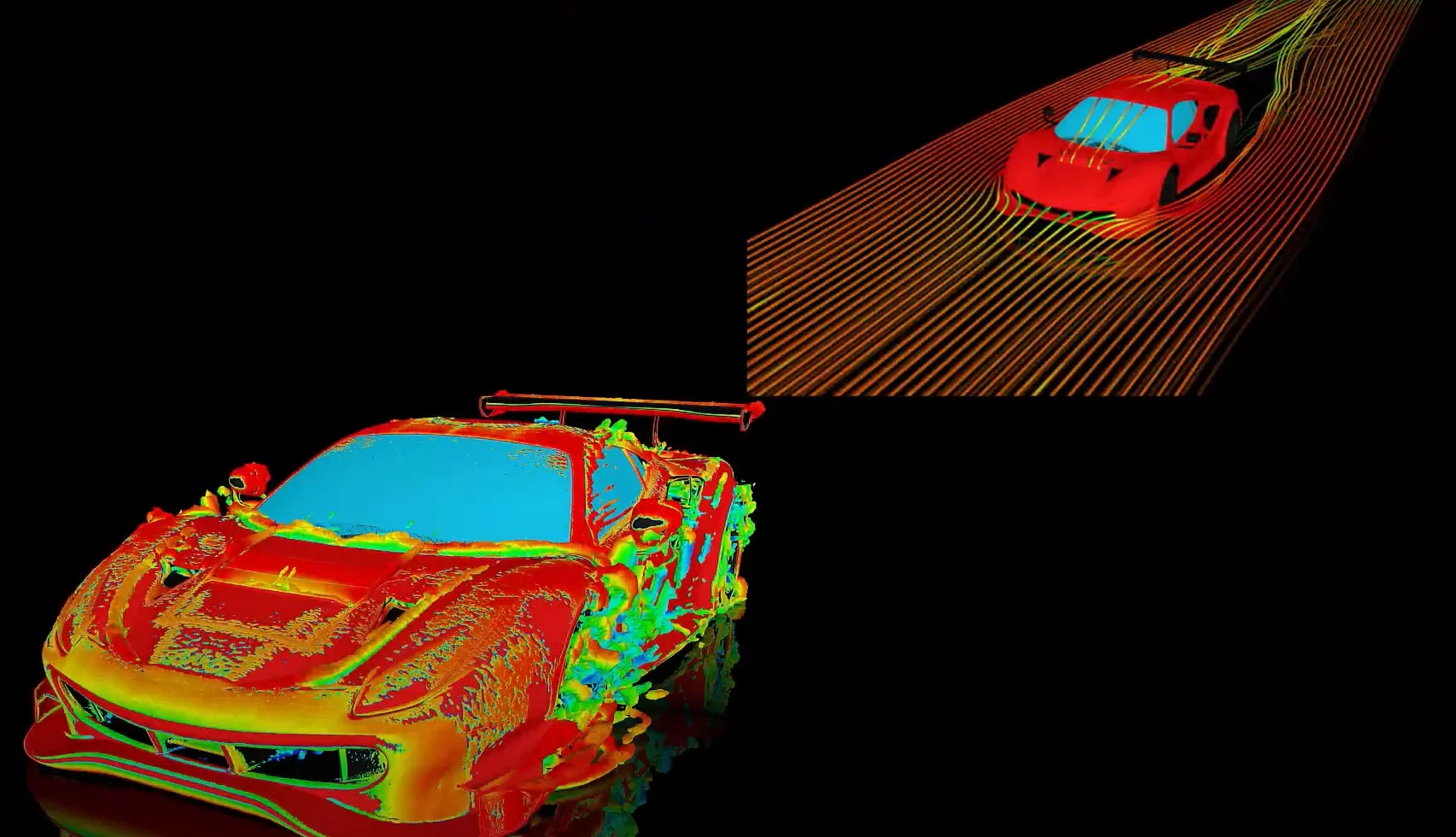
Introducing the GEKO Turbulence Model in Ansys Fluent
The GEKO (GEneralized K-Omega) turbulence model offers a flexible, robust, general-purpose approach to RANS turbulence modeling. Introducing 2 videos: Part 1 provides background information on the model and a...
Postprocessing on Ansys EnSight
This video demonstrates exporting data from Fluent in EnSight Case Gold format, and it reviews the basic postprocessing capabilities of EnSight.

- How to setup Initial, Minimum and Maximum time step in Transient analysis?
- How to define variable thickness shell elements in ANSYS Mechanical? Is there any verification example of the variable thickness shell modal analysis available?
- In Workbench Mechanical, how can I obtain strain energy output for Modal analysis?
- In a random vibration analysis, what is “GRMS” and how can it be calculated?
- Why is damped frequency is lower than undamped frequency with viscous damping but larger with structural damping?
- How to apply application-based settings to improve the performance and robustness of transient structural analyses?
- How to include effect of bolt pretension in a modal analysis?
- In the results of a modal analysis, how can I define that a frequency is an output parameter ?
- Presentation on shock analysis using response spectrum and transient dynamics
- How can I specify acceleration at a node? Could I use the ‘big mass method’?

© 2025 Copyright ANSYS, Inc. All rights reserved.

