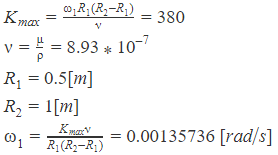Tagged:
-
-
September 26, 2022 at 10:00 am
 SolutionParticipant
SolutionParticipantThis example is taken from Cornell University’s ANSYS AIM Learning Modules
Problem Specification
A viscous fluid is between two concentric cylinders of radii a and b, which are rotating at constant angular velocities. The diagram below shows these two cylinders and their respective angular velocities. In this problem, the velocity of the inner wall must be calculated to create the Taylor-Couette phenomenon. Find the velocity vectors that are characteristic of the Taylor-Couette flow.
Pre-analysis
- In the paper “Instability of Taylor-Couette Flow between Concentric Rotating Cylinder” by Hua-Shu Dou, Boo Cheong Khoo, and Khoon Seng Yeo the equation for the critical condition of primary instability is simplified for a concentric rotating cylinder. It is given and solved in the equations below. In order to incite Taylor-Couette flow, Kmax must be between 370-389 so a value of 380 was chosen as for Kmax the calculation.
Geometry & Mesh
In this video, you will learn how to create the geometry in Discovery AIM how to generate mesh in the computational fluid domain.
Physics Setup
In this video, you will learn how to edit material properties and specify fluid flow conditions.
Results Evaluation
In this video, you will learn how to evaluate velocity vectors and Pressure contour in the flow domain.
Reference
Dou, H.-S., Khoo, B.C., and Yeo, K.S., Instability of Taylor-Couette Flow between Concentric Rotating Cylinders, Inter. J. of Thermal Science, Vol.47, 2008, Vol.47, No.11, 1422-1435.
-


Introducing Ansys Electronics Desktop on Ansys Cloud
The Watch & Learn video article provides an overview of cloud computing from Electronics Desktop and details the product licenses and subscriptions to ANSYS Cloud Service that are...

How to Create a Reflector for a Center High-Mounted Stop Lamp (CHMSL)
This video article demonstrates how to create a reflector for a center high-mounted stop lamp. Optical Part design in Ansys SPEOS enables the design and validation of multiple...
Introducing the GEKO Turbulence Model in Ansys Fluent
The GEKO (GEneralized K-Omega) turbulence model offers a flexible, robust, general-purpose approach to RANS turbulence modeling. Introducing 2 videos: Part 1 provides background information on the model and a...
Postprocessing on Ansys EnSight
This video demonstrates exporting data from Fluent in EnSight Case Gold format, and it reviews the basic postprocessing capabilities of EnSight.

- Discovery Live: How to change units
- Discovery AIM tutorial – Analysis of cantilever beam with I cross-section
- Discovery AIM tutorial – 3D Finite-Element Analysis of a Bike Crank
- Discovery AIM – Scaling deformation contour results
- FAQ: Missing Areas of the User Interface in Discovery Live
- Discovery AIM tutorial – Structural Analysis of Plate with Hole
- Discovery SpaceClaim: Getting Started Tutorials
- Creating a Helical Geometry in SpaceClaim
- How to setup a Parameter Study
- Convert 2D Drawings to 3D Models in Minutes with SpaceClaim

© 2025 Copyright ANSYS, Inc. All rights reserved.