Why the analysis takes multiple iterations to solve even it has bonded contact and linear in nature?
Tagged: 19, General, mechanical, structural-mechanics
-
-
April 5, 2023 at 2:32 pm
 FAQParticipant
FAQParticipantThere is a list of features that trigger Newton-Raphson iterations (i.e., a nonlinear solution), but having any contact elements (even if bonded) will trigger NR iterations. (The other things that trigger NR iterations – plasticity, nonlinear springs, etc. – are usually more obvious.) What we do in Workbench Mechanical is to use the following command to force a single iteration:neqit,1,force With this, one will see the following warning printed: *** WARNING *** CP = 2.090 TIME= 12:09:08 Using 1 iteration per substep may result in unconverged solutions for nonlinear analysis and the program may not indicate divergence in this case. Check your results. What this warning is saying is: if the analysis is truly linear (i.e., small deflection theory), you should have force balance; if the analysis is actually nonlinear, you won’t get force balance. Now, let’s say the analysis was solved as a ‘linear’ analysis, but large deflection effects should have been turned on – we wouldn’t know this, either, since a ‘linear’ analysis doesn’t use NR method. Hence, by specifying “neqit,1,force”, we are saying that we believe that it’s a linear analysis, and we are forcing that assumption on the solver.If you want to be safe, then use 1 substep (NSUBST,1,1,1), but this may require multiple equilibrium iterations. If it’s linear, it should converge quickly (e.g., 2-3 iterations), and one will be ensured of numerically accurate results.If one is confident that the analysis should be run as linear, then use 1 equilibrium iteration (NSUBST,1,1,1 with NEQIT,1,FORCE) and there will not be any additional checks, but the solution will really just be 1 iteration, like a linear analysis.
-


Introducing Ansys Electronics Desktop on Ansys Cloud
The Watch & Learn video article provides an overview of cloud computing from Electronics Desktop and details the product licenses and subscriptions to ANSYS Cloud Service that are...

How to Create a Reflector for a Center High-Mounted Stop Lamp (CHMSL)
This video article demonstrates how to create a reflector for a center high-mounted stop lamp. Optical Part design in Ansys SPEOS enables the design and validation of multiple...
Introducing the GEKO Turbulence Model in Ansys Fluent
The GEKO (GEneralized K-Omega) turbulence model offers a flexible, robust, general-purpose approach to RANS turbulence modeling. Introducing 2 videos: Part 1 provides background information on the model and a...
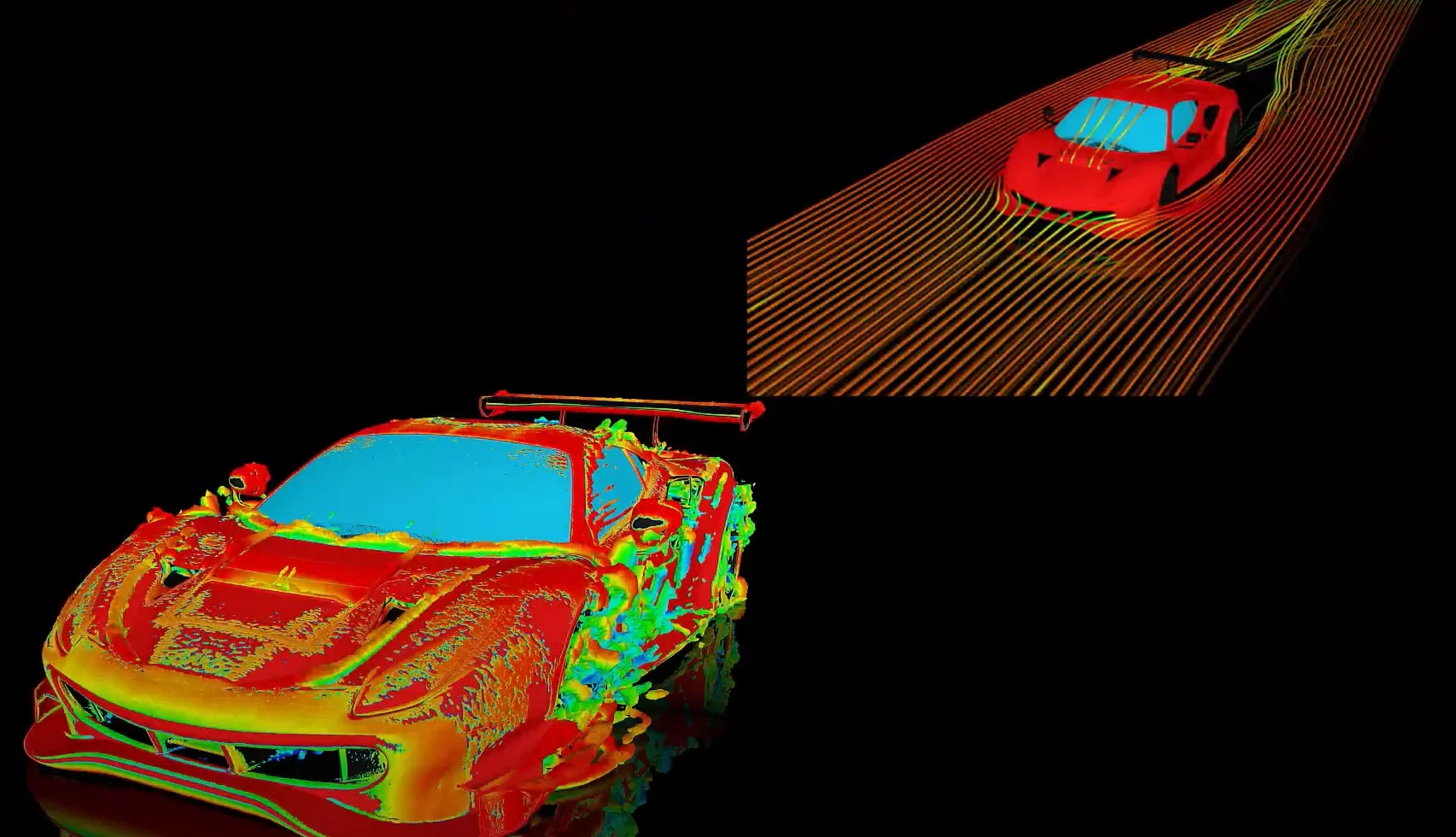
Postprocessing on Ansys EnSight
This video demonstrates exporting data from Fluent in EnSight Case Gold format, and it reviews the basic postprocessing capabilities of EnSight.

- How do I request ANSYS Mechanical to use more number of cores for solution?
- Contact Definitions in ANSYS Workbench Mechanical
- How to restore the corrupted project in ANSYS Workbench?
- How to deal with “”Problem terminated — energy error too large””?”
- There is a unit systems mismatch between the environments involved in the solution.
- How can I change the background color, font size settings of the avi animation exported from Mechanical? How can I improve the resolution of the video?
- How to transfer a material model(s) from one Analysis system to another within Workbench?
- What is the reason for this error message when mesher fails – “A software execution error occurred inside the mesher. The process suffered an unhandled exception or ran out of usable memory.”?
- How to obtain force reaction in a section ?
- How to change color for each body in Mechanical?

© 2025 Copyright ANSYS, Inc. All rights reserved.

