TAGGED: cavity, photonics, ring-resonators, varFDTD, waveguides
-
-
January 14, 2022 at 2:51 am
cflower
SubscriberHi,
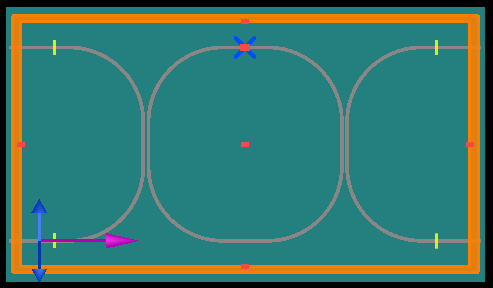
I am attempting to simulate a racetrack resonator in an add-drop filter configuration (so with two coupled waveguides.) Full 3D FDTD is too demanding, so I have been using the 2.5D varFDTD solver in MODE.
The device parameters are the following: SiN (sampled index file) waveguide, 1um wide by 800nm tall, embedded in SiO2 (index = 1.46). The bending radius of the round sections of the couplers and resonator is 20um. The straight sections are all
12um. The coupling gap is 400nm (but this could be anywhere from 300 to 800nm). I have placed four transmission monitors at the input, through, add, and drop ports, and I am exciting the input waveguide with a mode source set to fundamental mode. I would like to see the transmission from approximately 1540nm to 1560nm. The varFDTD slab mode position is set to one of the racetrack straight segments.
The simulation runs fine, but the Free Spectral Range (FSR) is way off. In experiment, I have consistently observed an FSR of approximately 3nm for this device, but the simulation consistently gives me something approximately twice that.
In the past I have simulated similar (albeit smaller) devices with Silicon waveguides and have managed to replicate experimental results. The exact simulation parameters used there (such as meshing, sim time, varFDTD effective index settings etc) are not giving correct results for the SiN system.
I am aware that broadband simulations in 2.5D can be an issue. I have the varFDTD object set to a broadband simulation, but I can't rule out that something is breaking there because the mode source is single wavelength.
Please let me know if there are any other settings that might be an issue, I am running out of ideas to try and replicate these results.
I have attached some screen shots and am happy to upload an .lms file. Thank you very much for any help.
January 14, 2022 at 5:47 pmGuilin Sun
Ansys EmployeeThis is an interesting question.
varFDTD is a variation to the original 3D FDTD. It can perform the best when the decomposed 1D modes well match 2D modes: it decomposes the original 2D modes into 1D z lab mode in "effective index" tab, and the mode source (in your case it seems 1D y equivalent waveguide). Its physics can be found here MODE - 2.5D varFDTD solver introduction
and it is know that FSR is different due to the decomposition: https://support.lumerical.com/hc/en-us/articles/360042800293-Ring-resonator-getting-started-Design-and-initial-simulation
It is used for quick design but we recommend to use 3D FDTD for final verification.
In addition, please make sure that the SiN material data in the material database represents your actual device material. Otherwise your design will deviate the real device. As you can seem the material data in the material database has large difference between Phillip and Kischkat .
As for the discrepancy of neff, it is normal because of the waveguide is "different" (check the cladding effective index). However, when you use "user select", please make sure it is the same polarization as in the "Effective index". This is critical to get correct result.
One more thing: You do not need to use single wavelength source. I would suggest to use broadband, as you mentioned from 1.54 to 1.56um would be better, since numerical dispersion will shift the actual resonance. (This is a different topic).
January 14, 2022 at 7:19 pmcflower
SubscriberHi gsun
Thanks for your reply! I think what you said about the material data may be crucial. In particular I recreated the waveguide geometry cross section in another file (using the same material files) and calculated the group index for the fundamental mode. It is very close to 2.075 across the whole wavelength range.
I then did a basic calculation. FSR = c/(L * ng) where c is speed of light, L is the length of the resonator (4*12um+2pi*20um=173.7um).
This gives FSR = 0.83 THz, which is exactly what I observe in simulation, which disagrees with experiment. I think this is a good indication that 2.5D is creating a 2D model that accurately represents the group index that Lumerical has calculated, but this group index does not correspond well to experiment. The only reason I can think of that would cause this is that the material file is inadequate.
I am attempting the same calculation with the Kischkatmaterial file now. I will update here if it ends up replicating the measurement well. It is very strange that there is so much disagreement between material files for SiN... I wonder why that is.
January 14, 2022 at 7:28 pmGuilin Sun
Ansys EmployeeComparing simulation with experiment can be challenge in some cases, especially if you do not know exactly the material the device used. Please refer this post:
Ansys Insight: Why my simulation result is different from published paper or experiment?
January 14, 2022 at 9:07 pmcflower
SubscriberHi gsun, thank you for that link. I will go through it.
In this case, I performed the experiment so I have very detailed knowledge of the device in question. Using the Kischkat material file did not produce the correct results. So far I have 3 different FSRs: 0.83 THz from our sampled material file, 0.65 THz from Kischkat, and 0.4 THz from experimental measurement.
I believe the problem is in the calculation of the group index. I attempted to calculate the group index with "detailed dispersion calculation" on but saw no change. I am now using the multi-coefficient model to get a better fit to the sampled data.
I will keep trying this as well as things in the list you provided.
January 14, 2022 at 11:20 pmGuilin Sun
Ansys EmployeeI would suggest to have a measured refractive index from your device, as the material can have quite different property. The group index is correct from FDE but it depends on the material index (and waveguide).
January 17, 2022 at 6:14 pmcflower
SubscriberHi gsun The material file I have is a measured/sampled refractive index file from the foundry that performed the fabrication. It should be reliable. Selecting "detailed dispersion" calculation and fine tuning the multi-coefficient model made no significant changes to the calculated group index.
I am currently performing some 3D FDTD simulations of the ADF to see how the FSR comes out. The rings themselves are very large though (20um bending radius + 12um straight sections) so I may not be able to simulate the system beyond a coarse mesh (mesh accuracy 2, possibly 3 at best...). If it replicates the 2.5D result we can probably assume that the issue is either the index file or the device itself not being to spec (which seems unlikely).
If it does not replicate the 2.5D, there are a few possibilities:
1. Any difference in meshing may have caused an issue.
2. The source's frequency dependent profile option in FDTD that is not available in MODE made a difference.
3. The effective 2D index calculated by MODE is not accurately representing the 3D geometry for some reason.
If the simulation fails to finish in a reasonable time, I will simulate a smaller ring. It will not agree with our experiment, but we can at least test if 3D agrees with 2.5D for this material file.
January 17, 2022 at 7:16 pmGuilin Sun
Ansys EmployeeI do not expect that 3D agrees with 2.5D, as explained previously, especially the FSR. This is because the waveguide is simplified, and since the neff is different from FDE (2D cross section) from two 1D waveguide, the resonance can be different. Smaller FSP means strong resonance, so you will need
1: significantly reduce autoshutoff min ,
2: increase simulation time.
You can take advantage of "check point": set long enough simulation, change autoshutoff min from one simulation to resume with smaller autoshutoff min. Please refer
https://support.lumerical.com/hc/en-us/articles/360036896474-resume-Script-command
https://support.lumerical.com/hc/en-us/articles/360034382534-FDTD-solver-Simulation-Object
January 17, 2022 at 7:32 pmcflower
SubscriberHi gsun Sorry if I missed that, but that is very surprising to hear... even the 2.5D whitepaper ( https://www.lumerical.com/learn/whitepapers/lumericals-2-5d-fdtd-propagation-method/) uses an add-drop config ring resonator as its main example.
They even explicitly say "In particular, the key quantities we typically extract from such a simulation, the bandwidth and free spectral range (FSR), are very accurately calculated with the varFDTD method, which is not the case with the standard 2D FDTD approach." indicating that varFDTD can accurately recreate 3D results for the FSR (albeit shifted, which is fine).
I do not see how my simulation is any different than this example, with the exception of the material and straight sections of the ring. In fact, I have been able to replicate experiment just fine with 2.5D previously in silicon (not silicon nitride) devices.
Thank you for the information on using a checkpoint, that could be very usful.
January 17, 2022 at 8:12 pmGuilin Sun
Ansys EmployeeWhether varFDTD can accurately get the result the same as 3D FDTD pr not strongly depends on the device. Please refer the reference papers listed in the website. In addition, please refer the exam[le O sent previously. Specifically for this result:
 The white paper indicates varFDTD is much better than 2D FDTD, not 3D FDTD! Please read the text again.
The white paper indicates varFDTD is much better than 2D FDTD, not 3D FDTD! Please read the text again.
varFDTD is a quick yet accurate method. However it has limitations and its accuracy needs to be VERIFIED with 3D FDTD, with sufficient long simulation time to let the time signal with high Q resonance completely decay.
I think I have said enough about the difference. between varFDTD and 3D FDTD. However the difference between simulation and experiment can be originated from many different causes. Please read the post previously provided.
January 17, 2022 at 8:31 pmcflower
SubscriberHi gsun
Thanks for responding again. I did not say that varFDTD is better than 3D at any point. I said "varFDTD can accurately recreate 3D results for the FSR" which is the claim the white paper is making about that particular simulation. I believe I have a good understanding of the limitations of varFDTD compared to full 3D FDTD.
I will look at some of the papers referenced on the website.
January 18, 2022 at 1:32 amGuilin Sun
Ansys Employeeok. the statement "varFDTD can accurately recreate 3D results for the FSR" is without problem. However extending its meaning to 3D FDTD might be problematic. 3D results for the FSR means it counts for the 3rd dimension. But it can be different from 3D FDTD. That is what I understand. I personally do not expect to have the same FSR from varFDTD as the 3D FDTD.
When the physical device is fixed, from this
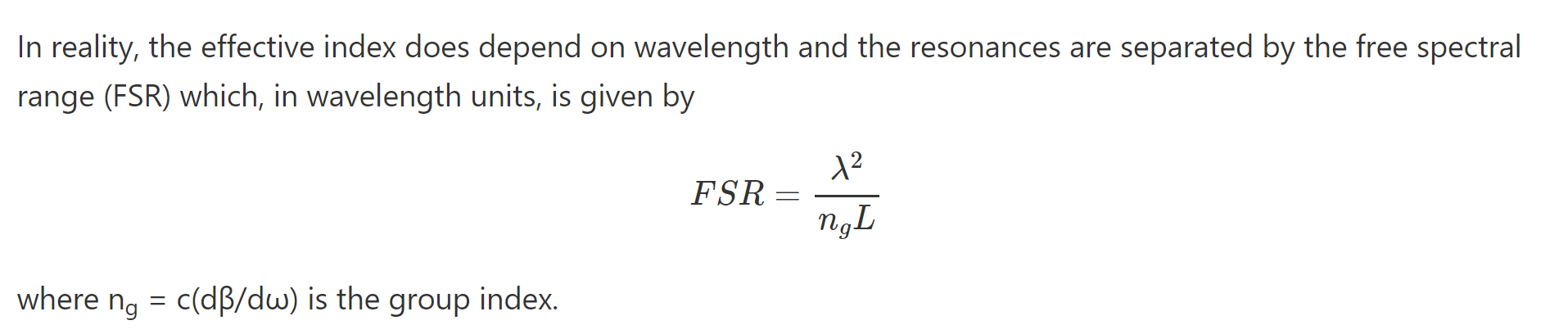 The resonant wavelength is different between varFDTD and 3D FDTD, I do not think the FSR is the same, except a coincidence that lambda^2/ng is fixed for the two simulations.
The resonant wavelength is different between varFDTD and 3D FDTD, I do not think the FSR is the same, except a coincidence that lambda^2/ng is fixed for the two simulations.
April 29, 2022 at 4:44 pmcflower
SubscriberI am returning here to clarify what the solution was for future readers.
There are three values for the FSR in question:
Experimentally Measured FSR: 0.4THz
FSR Simulated with 2.5D varFDTD (MODE): 0.8 THz
FSR Simulated with full 3D FDTD: Unknown
The issue I described of course is that 1 and 2 did not match each other.
It turns out that the reason this was happening was because the real device experimentally was supporting both a TE and TM polarization. It just so happened that they were roughly shifted by about half the FSR, had a very similar FSR, and similar amplitude. This made the FSR appear to be about half of the actual FSR for the TE or TM mode only, hence the measurement being off the simulation by a factor of two.
2.5D varFDTD was only able to simulate the TE mode, so we saw the full FSR at 0.8 THz.
I confirmed this by running 3D FDTD and rotating the polarization of the source to excite both TE and TM modes. Sure enough, there are two sets of modes, each with FSR about 0.8THz and shifted by about half an FSR, giving an apparent FSR of 0.4THz.
So all in all, the simulations agreed, 2.5D varFDTD just could not account for the second (TM) polarization.
Regarding the previous comment by gsun, it is true the resonant wavelength is slightly different between varFDTD and 3D FDTD, but the difference is very small and no where near accounting for a factor of 2.
April 29, 2022 at 7:41 pmGuilin Sun
Ansys EmployeeThank you for clarifying! As for 3D FDTD and varFDTD, only when lambda^2/ng is about the same for the same device length can they agree each other. and in this case it seem they are very close.
Your clarification once again confirms that: simulation can only duplicate the experiment result when the parameters and conditions are the about the same.
Viewing 13 reply threads- The topic ‘Simulating racetrack resonator add-drop filter with MODE 2.5D – Getting incorrect FSR’ is closed to new replies.
Ansys Innovation SpaceTrending discussions- Difference between answers in version 2024 and 2017 lumerical mode solution
- Errors Running Ring Modulator Example on Cluster
- INTERCONNECT – No results unless rerun simulation until it gives any
- Import material .txt file with script
- Trapezoidal ring
- Help for qINTERCONNECT
- Absorption cross-section of AuNR excited by prism-based TIR
- Issues with getting result from interconnent analysis script
- How to measure transmission coefficients on a given plane .
- Topology Optimization Error
Top Contributors-
1416
-
599
-
591
-
565
-
366
Top Rated Tags© 2025 Copyright ANSYS, Inc. All rights reserved.
Ansys does not support the usage of unauthorized Ansys software. Please visit www.ansys.com to obtain an official distribution.
-