Learning Forum

[Cornelius Bong]

Hi, I'm new to Ansys. I currently using transient structural to study the effect of rolling speed in web handling roll-to-roll process. I try to copy the flat belt simulation available in youtube. The solver engine was unable to converge on a solution for the nonlinear problem as constrained. How can I solve this error. Thank you.

[peteroznewman]

Hi Cornelius, there is much to learn to get a nonlinear model to converge. Please use File Archive to save a .wbpz file. Put that file (not the .wbpj file) on a file sharing site like Google Drive or OneDrive. Make sure to set the permissions so that anyone can download the file. I will take a look.

There are tutorials on nonlinear convergence and several questions on the forum on this topic.

/courses/index.php/courses/contact-mechanics/

/courses/index.php/courses/large-deformation/

Here is a Google Search result for the phrase “unable to converge” in this site, but nothing older than 3 years.  Some older results have broken links that will land you on the home page.

[Cornelius Bong]

 

Hi, here is the file for the model.

https://unimas-my.sharepoint.com/:f:/g/personal/72168_siswa_unimas_my/Em3UjgVWX3pIp4uqGUzoyZABifpGlgjQ0n677b2zLvESTg?e=WqPnHq

What I try to do is to investigate the effect of rolling speed to the stress and deformation of the web. I set 5 number of steps and apply joint rotational velocity of 2.5, 5, 7.5, 10 and 12.5 rad/sec.

Thank you

 

[peteroznewman]

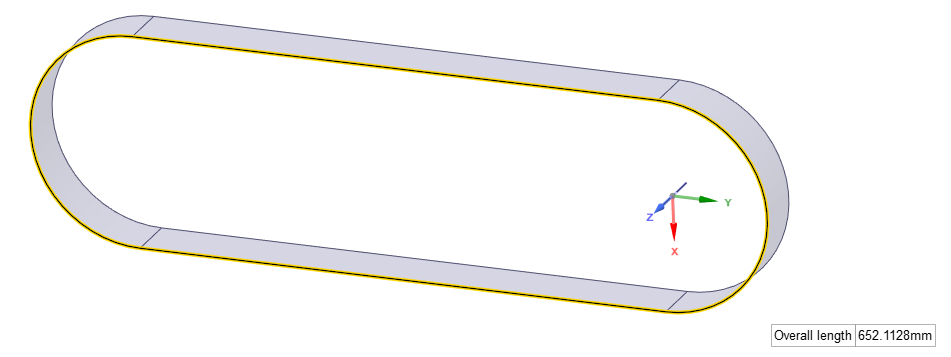
Belts are made circular.  When they are installed on a pair of pulleys, they are stretched out of the circular shape and into the "racetrack" shape.

The simulation for this model assumes a belt was manufactured in this shape:

If you turn the pulley in this simulation, a section of material that was manufactured curved has to be bent straight and a section that was manufactured straight has to be bent. You will see the curved sections of belt be transported between each pulley. That will be slightly amusing but will not reflect real world behavior. The state of stress of the belt will depend on the angle of the pulleys.

The proper way to simulate the behavior of a circular belt is to take 2 pulleys that are tangent to the circular belt and move one pulley until it stretches the belt into a racetrack shape. Only then do you want to rotate one of the pulleys to move the belt. In that way, the state of stress of the belt will be independent of the angle of the pulleys.

An exception to this logic is if the web is thin so it can be assumed to have no bending stiffness and only in-plane (membrane) stiffness. Then you can draw the web in the shape of a racetrack, turn a pulley and the state of stress in the web will be the same at any pulley angle.

How do you want to proceed?

[Cornelius Bong]

Based on what I understand from your comment, the geometry is the reason for error to occur in the simulation. What modification should be made to the geometry to solve the error?

 

How to model the geometry based on the suggestion below?

”The proper way to simulate the behavior of a circular belt is to take 2 pulleys that are tangent to the circular belt and move one pulley until it stretches the belt into a racetrack shape.”

 

Thank you

 

[peteroznewman]

I didn’t work on getting that geometry to converge. I wanted to hear back from you on whether the web that you want to transport can be assumed to have no bending stiffness. This could be a simplifying assumption for very thin web thickness.  Thick belts as as found in machinery are circular when manufactured and a racetrack as the undeformed shape would be silly. What is the web thickness you want to simulate and what is the Young’s Modulus and Poisson’s Ratio of the web material?

To take a circular belt and stretch it into a racetrack shape, take two pulleys tangent to the circle and move one to the stretched position in step 1 of a multi-step solution.  Step 2 can rotate the fixed pulley.

[Cornelius Bong]

I think the web in the simulation for my investigation can assume to have no bending stiffness.

The web thickness that I want to simulate is 0.05mm and the web material is PET plastic that is available in Ansys engineering data.

The Young' Modulus is 2.898e09 Pa while the Poission's Ratio is 0.3887.

[peteroznewman]

Okay, there must be some tension in the web and we want gravity turned on. Gravity is created using an Acceleration load. Accelerating in the +X direction causes mass to experience a downward force in the same way as if Standard Earth Gravity was set to the -X direction. The benefit of an Acceleration load over using Standard Earth Gravity is the magnitude of acceleration can be ramped up from zero using an Acceleration.

Note that a Joint Moment load was added to provide a load to turn against and create higher web tension in the top (+X side of the web) than the bottom.

The web has Membrane Stiffness only as previously mentioned.

Tension is created by an offset in the contacts, which is resolved in Step 1, with Time Integration Off.
Note that the Normal Stiffness Factor is 0.1 which helps the contact algorithm converge.

Gravity and the Moment load are ramped on in Step 2 with Time Integration Off. This concludes the setup of the model.

Step 3 is where the rotational velocity ramps up to 1 rad/sec.

Here is the Ansys 2022 R2 archive:  https://jmp.sh/R3keBHOx

[Cornelius Bong]

Thank you very much. This helps me a lot.