Learning Forum

[peteroznewman]

Thanks for the pictures Emir, that really helps me to understand your setup.

You show E.xy but that is not an entry for an Orthotropic material and I don't know what that means, perhaps that is G.xy?. 
Poisson ratio has no effect on the natural frequency of shell elements using Isotropic materials, based on a test in ANSYS.

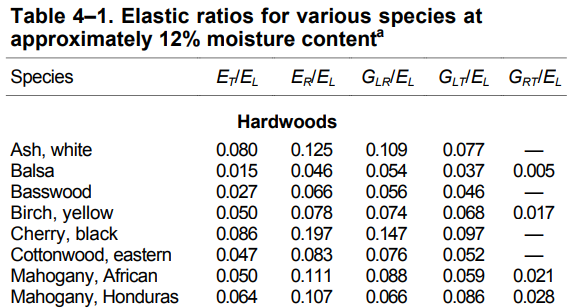
I found a paper that reports the Othotropic elastic properties of many types of wood. That paper uses the terms L, R and T to represent Longitudinal, Radial and Transverse directions in the wood. If you assign the x axis to Longitudinal, which axis is going to be Radial? Aren't most sheets of wood going to have the Radial direction be the thickness direction? Is thickness Z in your definition? I see that the Poisson Ratio in this paper is > 0.5 in many cases. That is because they have measured the shear modulus G. The paper also shows the magnitude of Young's Modulus relative to the Longitudinal axis (grain direction).

Based on this table, I would expect to see values of E.y and E.z to be a small fraction of E.x

The table for Poisson Ratio shows that there are different values depending on which direction is being stressed.
Note that the value for LR is not equal to the value for RL.

The Orthotropic material model in ANSYS can only handle the case where LR = RL.
I don't know how you can incorporate this data in ANSYS, maybe others will comment?

---

Here is a totally different idea: use Euler beam equations that only include the parameter EI to characterize the bending stiffness of the beam. You could use narrower strips of wood. As the strip gets narrower, the first mode will not be a twisting mode.  b can be much smaller than 182 mm for this idea.

The equation for the first natural frequency of a free-free Euler beam is:

where I searched for the value of E that would deliver the 65 Hz from your experiment.
When I type this value into an Isotropic material, ANSYS calculates a frequency of 65.1 Hz.
The shear modulus is automatically calculated for an isotropic material but does depend on Poisson ratio.

Say the first test had the grain running parallel to the length of the rectangle to measure E.x.
Cut and test another piece where the grain runs parallel to the width of the rectangle to get an estimate of E.y from its first natural frequency.

Create an Orthotropic material by using the same value as E.y for E.z since these directions are both across the grain. Use the values of Shear Modulus calculated by the Isotropic material. However, Shear Modulus is affected by Poisson Ratio in the Isotropic material. I would use the same value of Poisson Ratio for XY and XZ.

I recommend you carefully check the thickness is exactly 3 mm. If the thickness is off by only 0.1 mm, the value of E changes by nearly 7%

What do you think?

Regards, Peter