Reply To: Initial Velocity Generation

[valerym.m5]

Dear Pedram,

I will definitely take advantage of your kind offer.

But first I would like to make sure that my assumptions are correct.

 

So:

1. I could not learn to regulate the recording of calculation results at the initial stage of using an explicit solver.
For some unknown reason, the program ignores my instructions and writes the calculation results “arbitrarily” – immediately after switching the solver type – in this model – about 0.02 seconds.
After 0.02 seconds, the results are recorded with a step “close” to the required step, but still not exactly to my requirements specified in the curve.

 

 

 

•  I am interested in the calculation results at the initial moment of time – at the moment of impact of the load.

 

 

 

 

2. Since I couldn’t solve the 1st problem, I tried to use the command:
Velocity_Generation_Start_Time
Where, according to the documentation, you can set a “delay” in time for the initial velocity applied to the “rigid” body.
However, ls-dyna ignores any specified delay values ​​and the initial velocity is activated at the moment of switching from the implicit to the explicit solver.

 

3. Taking into account my unsuccessful attempts 1 and 2.
I decided to achieve the required delay in the time of application of the initial velocity by moving the falling load a distance that I calculated from the specified initial velocity of 1000 mm per second.
However, from the analysis of the solution I found that the initial velocity of the load is not 1000 mm per second, but 2567 mm per second!

0.00805664 sec is the time of motion of the rigid body
20.68639946 mm is the distance the rigid body has moved
2567.62117458 mm/sec is the constant speed of motion

 

If someone wants to get acquainted with my model – see the link below

https://drive.google.com/file/d/1jf3XCB1v87ImHqMO-PfN6SXNVcTPGAUT/view?usp=sharing

 

I will be very grateful for any advice – since in this case I have a stalemate.

———————————-

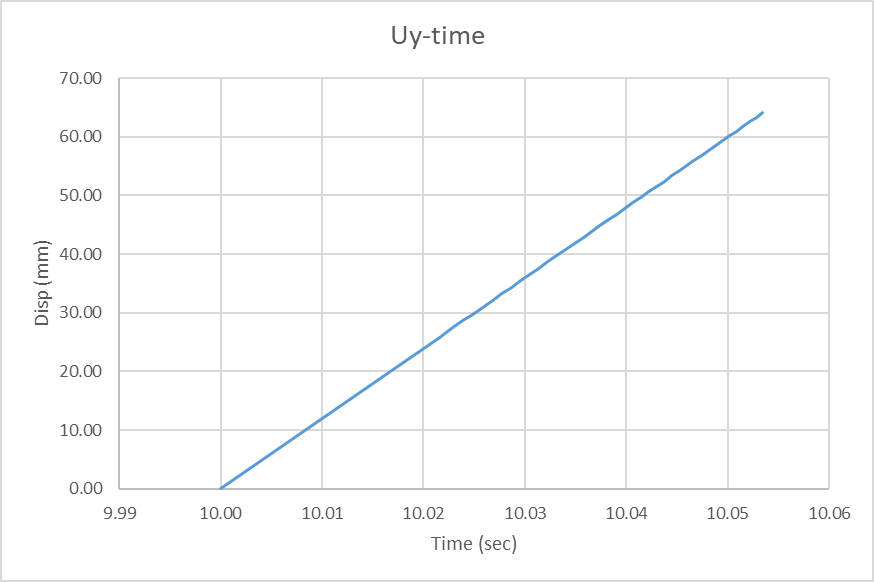
I tried to solve this problem with other data – trying to understand possible patterns, and I was very surprised, since the initial speed given was different each time?!

Below is another example of the solution

time	Uy
10.0000000	0.0000000
10.0168285	20.1738129
10.0215492	25.8324966
10.0228729	27.4195175
10.0238628	28.6061802
10.0248413	29.7792721
10.0258083	30.9385910
10.0267649	32.0844803
10.0277100	33.2173271
10.0286436	34.3369370
10.0295668	35.4437866
10.0304794	36.5377007
10.0313816	37.6189613
10.0322733	38.6876526
10.0331545	39.7442780
10.0340252	40.7885284
10.0348864	41.8205681
10.0357380	42.8409538
10.0365791	43.8493919
10.0374107	44.8462029
10.0382328	45.8315926
10.0390453	46.8054848
10.0398483	47.7680931
10.0406418	48.7197609
10.0414267	49.6601524
10.0422020	50.5898819
10.0429688	51.5088005
10.0437260	52.4171677
10.0444756	53.3149796
10.0452156	54.2024994
10.0459471	55.0797882
10.0466709	55.9469223
10.0473852	56.8037834
10.0480919	57.6509781
10.0487909	58.4884300
10.0494814	59.3161201
10.0501642	60.1341667
10.0508385	60.9428711
10.0515051	61.7423515
10.0521641	62.5323830
10.0528164	63.3134384
10.0534601	64.0852509

 

Dt	0.0534601
D_Uy	64.0852509
speed	1198.7487

For the last solution, the initial speed was specified -2800 mm per second,

but in reality – according to the calculation – the speed was 1198 mm per second