TAGGED: ansys-workbench, static-structural
-
-
April 17, 2021 at 8:44 am
TaoZhanping
SubscriberHi, I want to get the critical load for the structure below. This is a cylindrical shell,with both ends are connected with the shell through **** threads. The second page is the scheme in WB.Both The eigenvalue Buckling Analysis and the nonlinear buckling analysis have been done. nHere is the setting of the analysis:n1 the third and fourth page is the material property.And the connection in both is bonded.n2 the boundary condition is one end with fixed support. And the force is normal to all the faces.n3 In nonlinear analysis, the load is Linearly increasing from 0 to 60MPa in 10 steps, in which the time of every step is 1s.n4 The failure criterion is the equivalent stress(Von-mise).When the stress exceeds the limit(290MPa),the load this step is the critical load.nQuestion:n1 In eigenvalue Buckling, I get the critical load is 384MPa.But in nonlinear analysis, the result is only 42MPa. I want to konw why the results differ so much.n2 From the contour of the equivalent stress in fifth picture, the max stress appears in the connection between the end and the shell. The result is not in line with reality.How should I solve the problem?nThank you for your reply.n
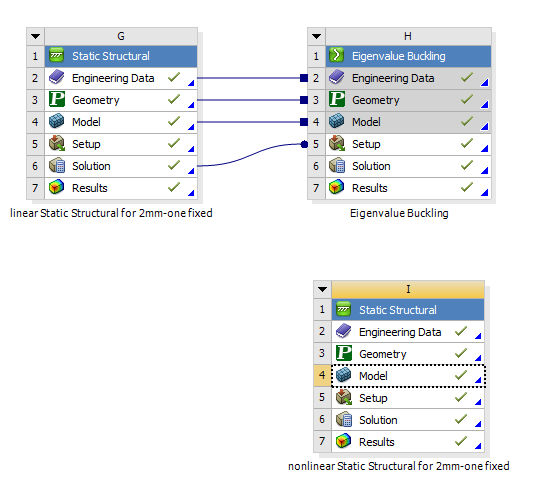
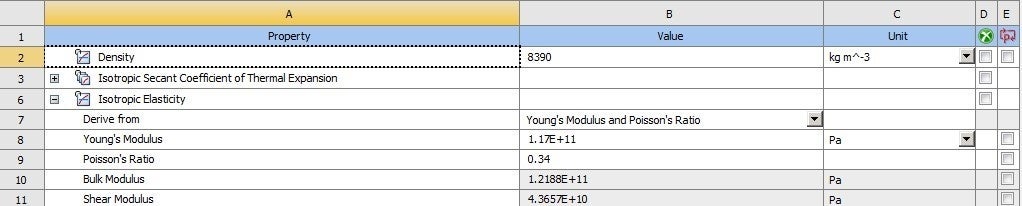
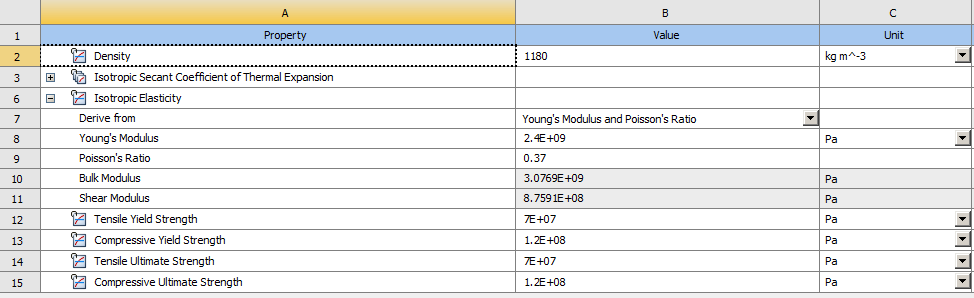
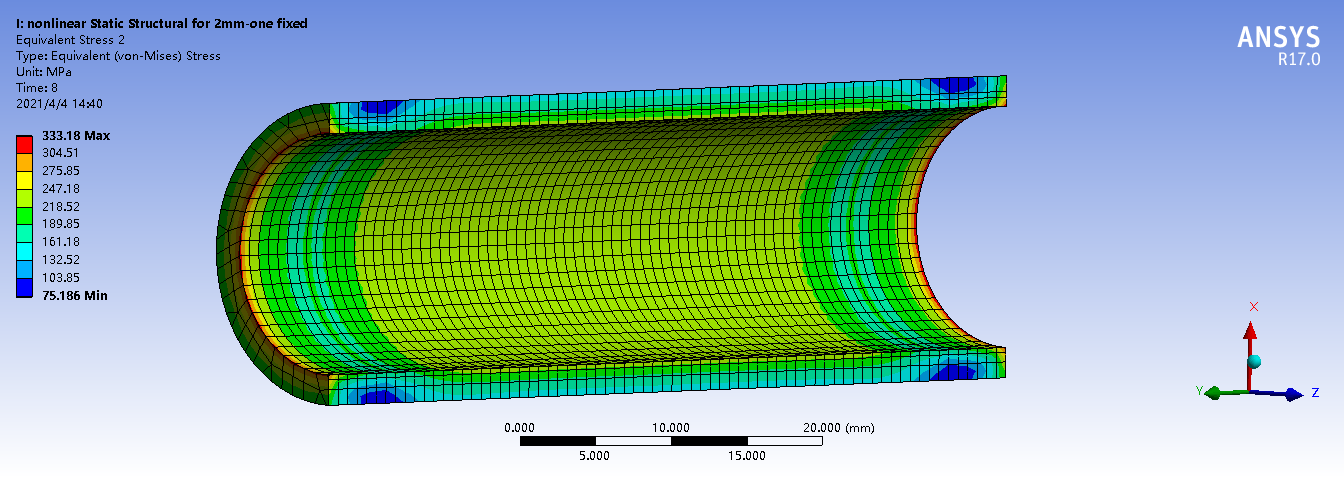
 n
n
-
April 20, 2021 at 11:53 am
1shan
Ansys Employee,nA compression member is usually subjected to two modes of failure 1. Material Failure (yielding) and 2. Buckling. Yielding is when a material crosses its elastic limit and deforms plastically. A von-mises stress criteria is used to predict failure of ductile materials by yielding. Whereas buckling is the sudden change in shape of a structure generally under axial compressive load(there are other modes of buckling as well). Buckling is characterized by a loss of stiffness of the structure, which is indicated by a horizontal force displacement curve in the non linear simulation , after which the solution fails to converge. Please have a look at which shows how to find the buckling point by tracking the load deflection curve. You need a tiny side load or deformation to initiate buckling. Also, It is better to apply a axial displacement instead of an axial force, this way, the solver does not fail to converge and you could see the buckling load increase to a maximum and then go down with a negative slo pe as structure continues to buckle.nRegards,nIshan.nn -
April 21, 2021 at 12:37 pm
TaoZhanping
SubscribernThanks for your conmment! In reality, I'm doing the test of hydrostatic pressure for the mode, which is surrounded with water. I want to get the similar result to the experiment in the simulation by ansys. From the egenvalue buckling,I can get the geometry fit to the experiment result. But in nonlinear analysis, when I set the load pressure a little higher than the egenvalue buckling ctrtical load ,I find the equivalent stress so much higher than the limit stress, which shows the yield or even fracture appear for a long time. nSo could you please give me some help to solve the problem?nThe following is the page aftrer the experiment and the critical load in test is 32-36MPa.nRegardsnTaon n
n
-
Viewing 2 reply threads
- The topic ‘The eigenvalue Buckling Analysis and the nonlinear buckling analysis’ is closed to new replies.
Ansys Innovation Space


Trending discussions


Top Contributors


-
2979
-
970
-
857
-
755
-
599
Top Rated Tags


© 2025 Copyright ANSYS, Inc. All rights reserved.
Ansys does not support the usage of unauthorized Ansys software. Please visit www.ansys.com to obtain an official distribution.







