Unsteady Flow over a Cylinder - Simulation Example
Overview
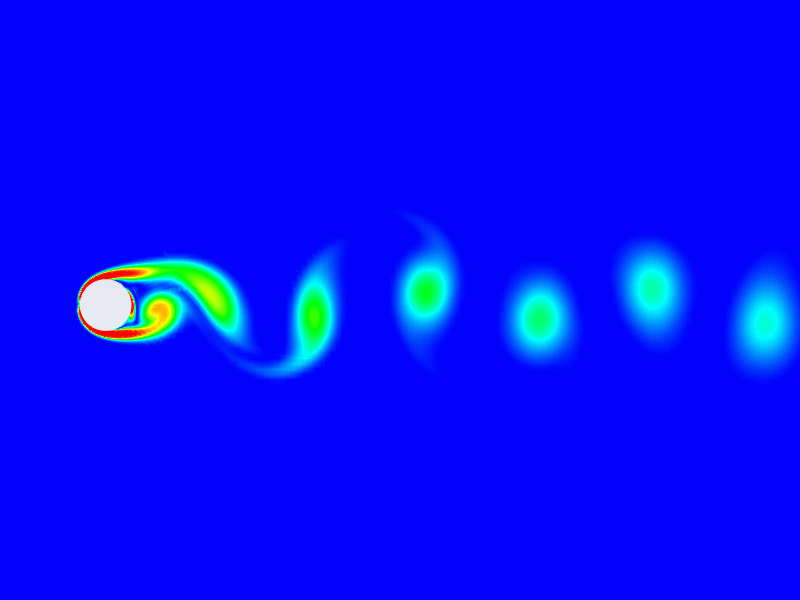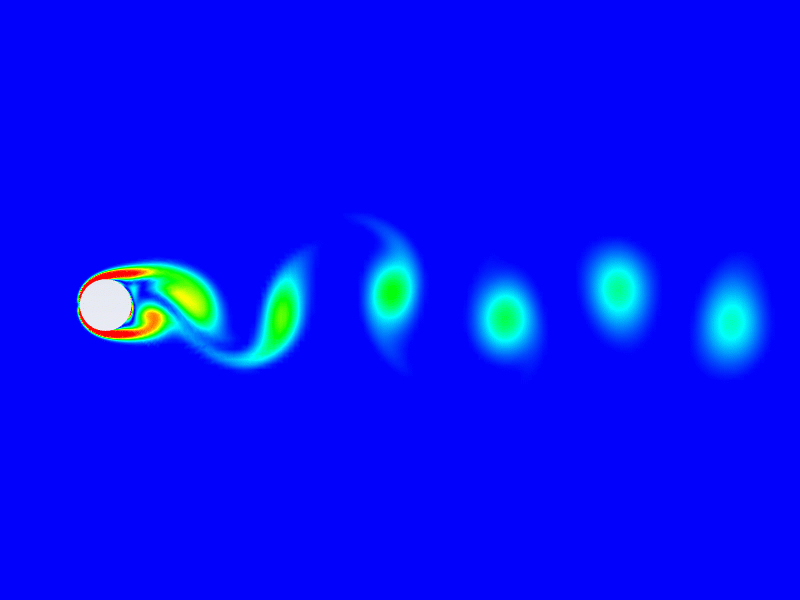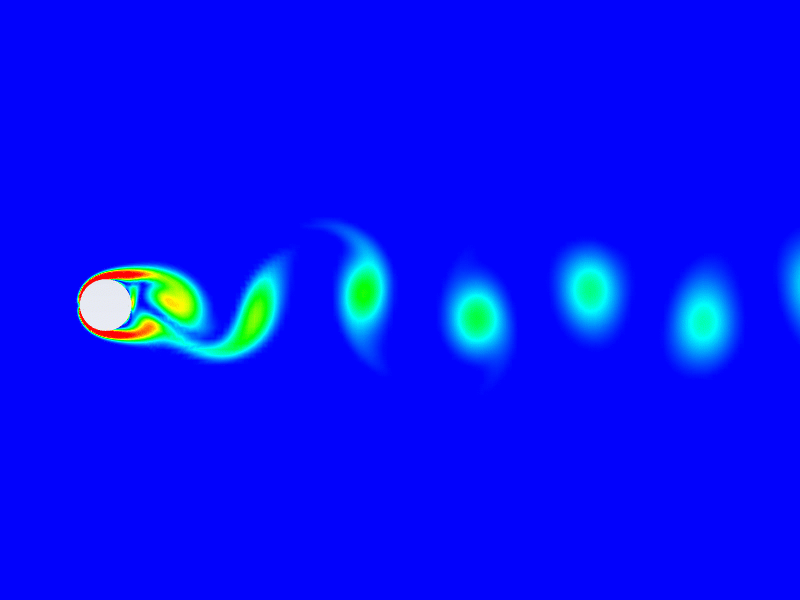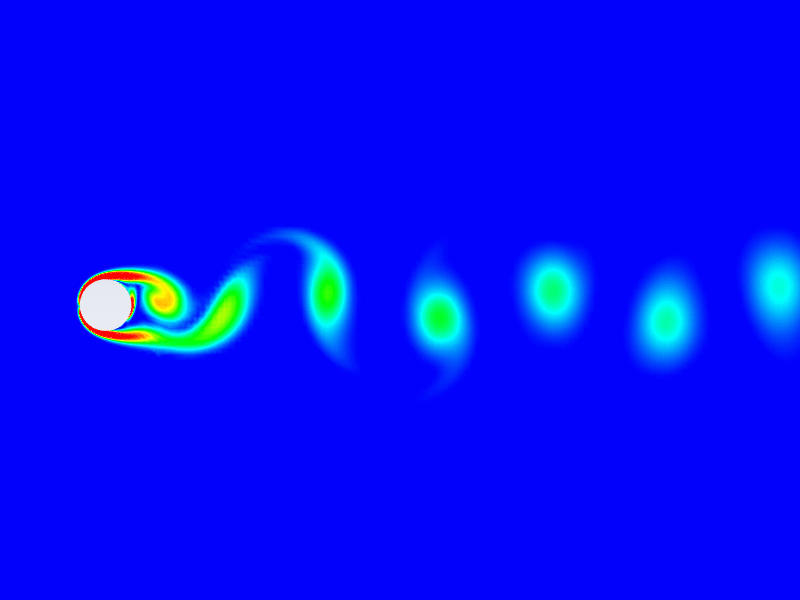
Physically, all fluid flows are naturally three-dimensional (3D) and therefore 3D models need to be used to analyze general fluids, especially those involving complicated geometries. However, in some cases proper assumptions can be made to reduce a 3D problem to two dimensions (2D) to simplify its analytical or numerical analysis. Generally, if the flow field gradients in one direction are much smaller than those in the other two directions, a 2D planar or axisymmetric assumption can be justified. For instance, a Stokes (or creeping) flow over a smooth sphere has zero gradients in the circumferential direction, so an axisymmetric assumption for the flow is appropriate. Another classic scenario is a Couette flow between two parallel flat plates, which can be approximated by a 2D planar model. In this example, a 2D simulation of an unsteady flow over a circular cylinder is performed. The 2D planar assumption is used because the flow gradients along the cylinder’s axial direction are negligible when compared to the other two directions. By reducing the model to 2D, the unsteady periodic vortex shedding process can be simulated and analyzed in a cost-effective manner compared to a full 3D model, and the results can be used to produce an animated visualization for the formation of a von Kármán vortex street.
Modeling Objectives
In this simulation example, you will learn:
- How to simulate a 2D unsteady flow over a circular cylinder
- How to create animations of flow variables in an unsteady flow
- Common methods to visualize vortices in a flow
Setup
Download the Mesh file needed for setting up the simulation and the associated Case & Data files from here. Follow the instructions below to set up this simulation in Ansys Fluent starting with a Mesh file. In case you face any issues setting up or running the simulation, then please use the corresponding initial and final Case and Data files.
Results and Discussion
Let us now analyze the simulation results and understand the physics of unsteady flow over a circular cylinder.
