Verification & Validation - Lesson 8

"Verification and validation" can be thought of as a formal process for checking results. Validation consists of assuring oneself that the solution is, in fact, correct. This consists of making sure the discretization error is minimized by performing a convergence study to assure oneself that the results are insensitive to the chosen mesh. This can be done by following similar steps taken in the 2D Beam Analysis tutorial here: 2D Beam - Verification and Validation

Once this is accomplished, one needs to compare the converged results with experimental data or directly applicable theory. In this case, we have experimental results for the deflection and simple Euler-Bernoulli theory for comparison with the stress results. The deflection results are obtained within the uncertainty of the measured tip deflection:

Performing the three-dimensional analysis results in tip deflections in agreement with theory including the shear deformation but does not render normal stresses at the wall given by simple beam theory.  Below are the hand calculations from (one-dimensional) Euler-Bernoulli beam theory and the predictions for the normal stress at the fixed wall obtained from a fully three-dimensional analysis that includes Poisson effects from the dimensions in the cross-section. Recall, from the calculation in the Pre-Analysis section, the normal stress at the wall due to bending deformation alone is 854.9 psi whereas that predicted by the FEA analysis is nearly double at 1715 psi.

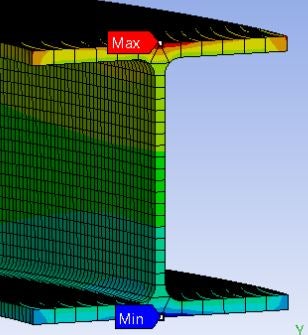
The Euler-Bernoulli theory predicts normal stresses in the absence of out=of=plane stresses (which are presumed negligibly small. Here the out-of-plane ZZ component of stress is predicted to be approximately 820 psi, i.e on the order of normal stresses due to bending alone. These substantial out-of-plane stresses result in Poisson effects on the normal wall stress, increasing its value substantially.